1. It is logically possible for a finite spatial region to evolve into a space that is both uniform and spatially infinite.
2. Inflation, our well-tested and widely accepted paradigm for understanding the very early universe, provides just the right physics and context for this to happen.
3. In the combination of our current best bets for cosmology and fundamental physics, inflation creates infinitely many “subuniverses,” each itself uniform and infinite.
4. This raises profound issues—for physics, cosmology, and even personal identity—that we cannot ignore.
1. A spatial region can evolve into an infinite, uniform space.
While this idea appears absurd, the apparent absurdity is based on intuitions about space and time that were profoundly undermined in 1905 by Einstein’s special theory of relativity.
A fundamental lesson of relativity theory is that there is no single, objective definition of what events are happening “now” across a large region. An observer can easily categorize nearby events into those that have happened, those occurring “now,” or those that might happen in the future. Yet faraway events cannot be immediately seen; information about them travels with, at most, the speed of light, so when a faraway object is observed, it is seen as it was some time ago. An observer must therefore infer, using a careful procedure, which events happened at the same time as a given event happening to the observer. What Einstein showed is that if two observers do this, and if the observers are moving with respect to each other, then they will make different inferences. According to one observer, two faraway events might happen at the same time; yet according to another observer, whose view is just as valid as the first, they happen at different times. More generally, given two events, different observers will ascribe different spatial distances, and different temporal intervals, between the two events (see Figure 1). What all observers will agree on, according to relativity, is the speed of light. This means that all observers will agree on whether or not an event is
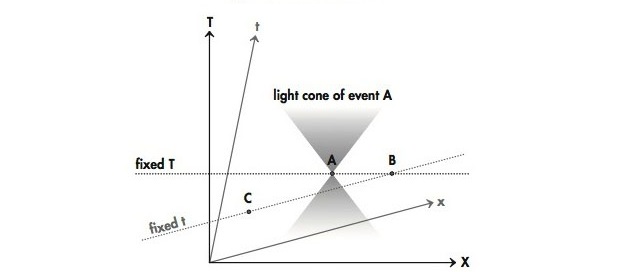
FIGURE 1: A space-time diagram of one spatial and one temporal dimension, in which light travels on diagonal paths. A given observer can label events by spatial coordinates X and times T, and describe all events at a given time T (shown as the “fixed T” line and including events A and B) as happening simultaneously. Yet a second observer, in motion with respect to the first, will label the same events by a different spatial coordinate x and time coordinate t, with all events at a given time t (indicated by the “fixed t” line) described as occurring simultaneously. Two events at the same time t (such as A and C) will not necessarily be at the same time T; that is, events such as A and B described as simultaneous for one observer will be described as happening at different times according to another observer. Both descriptions are equally valid. What all observers will agree on, however, is the speed of light. Thus if, for a given event A, we consider all regions of space-time that might communicate with A at sub–light speed, it constitutes the interior of a “light cone” (shaded region indicated) that all observers agree on. An event in the light cone of A can be in A’s past or future, but not simultaneous with A.
inside the light cone of another event (meaning that one event can influence the other event without transmitting information faster than light speed).
In relativity, then, space and time are interconvertible and should really be combined into “space-time.” To ask what “space” is like is really to ask what space-time looks like at a particular time. Yet special relativity says that space-time can be decomposed in many equally valid ways. According to Einstein’s general theory of relativity (1915), in fact, any two occurrences can be said to happen at the same time as long as a signal (or other causal influence) cannot be sent from one to the other—i.e., as long as one event is not within the other event’s light cone. Since special relativity forbids such influences from traveling faster than light, this criterion is equivalent to saying that the two events are outside of each other’s light cones.
As a fascinating and pertinent example of this freedom, consider plain, empty space-time—of just the sort we intuitively imagine: uniform, infinite, and uncurved, with the space looking the same in every direction and at all times. Yet we can divvy up this space-time in another very interesting way. We are free to define all events on a hyperboloid (see Figure 2) as occurring at the same time (even though this includes events that would, in the usual view, be considered as occurring at different times). This is allowed because no event on this surface can send a signal to any other event on this surface, as indicated by the light cones drawn in Figure 2. Moreover, each such hyperboloid can go on forever, without ever violating this constraint; thus, each comprises an infinite space. What’s less clear, but true if you go into the mathematics, is that each

FIGURE 2: A slice through one spatial dimension (plus time), of conventional space-time, with times indicated by gray lines and labeled by different values of T. At each time T1, T2, etc., space is infinite, uniform, and uncurved. Yet this space-time can with equal validity be decomposed into spaces—i.e., sets of events that happen at the same time—that consist of hyperboloids, as indicated by the black hyperbolas in the figure. Note that the light cone of any point on one of these hyperbolas does not intersect any other point on the same hyperbola. At each time t1, t2, etc., space is infinite and uniform but curved. In addition, all of these curved spaces fit inside the future light cone of a single point P.
such space is uniform: Just as flat space has constant zero curvature, or a sphere has constant positive curvature, hyperbolic space has constant negative curvature. Finally, what is really interesting about this construction is that the whole thing is contained within the future light cone of a single point. This point can therefore send a signal to anywhere in this infinite space without that signal’s ever exceeding light speed.
This construction directly undermines Kant’s argument; even though the space at any time t is infinite, one could imagine transmitting “assembly instructions” to the whole space specifying what properties it should have. And as we’ll see, this speculation is not an idle one.
2. Our best cosmological theories provide just the right physics and context for an infinite space to come into being through a physical process.
In order to apply his general theory of relativity to the universe, Einstein made the enormous assumption— beautifully justified since—that the universe on very large scales is essentially uniform. This allows only three geometries: the uniformly curved flat, spherical, or hyperbolic spaces mentioned above. Combining this with Edwin Hubble’s observation of cosmic expansion led to the Friedmann model of the universe: an expanding space of constant curvature. This model is the foundation of Big Bang cosmology, which correctly predicts the detailed evolution of the cosmos (reckoned to be 13.7 billion years old) from a simple initial state as a hot, nearly featureless medium of (mostly) hydrogen, helium, and dark matter.
Yet that initial state is itself puzzling in several ways. Perhaps most troubling is its uniformity. Uniformity in nature generally results from some smoothing process in which variations are evened out. Yet when we observe the early universe, we can see that it is uniform over scales far larger than such processes—which are limited by the speed of light—could possibly have operated across, if the Big Bang model held all the way back to a putative “beginning of time.”
Thus, a fix was devised. In the 1980s, cosmologists developed a theory called inflation, in which the very early universe expanded rapidly and exponentially, doubling in size scores of times in a tiny fraction of a second. This sort of expansion can be driven by so-called vacuum energy contained in empty space and has several crucial features. First, because space is expanding so quickly, any matter present is diluted to very low density and any waves are stretched out to form uniform fields; thus, the content of the universe looks homogeneous. Second, a small uniform region can expand so fast that the rate of change of the size of the region exceeds the speed of light. (This does not violate special relativity, which forbids signals traveling faster than light with respect to space-time itself but does not forbid objects from separating faster than light.) Third, positions far enough apart in inflating space cannot contact each other; because the intervening space is expanding so quickly, even a light signal sent directly from one location toward another will never arrive, as long as inflation continues. This defines a “horizon” about a given position, from beyond which no influence can be felt.
These three aspects are what allows inflation to ameliorate the peculiarities of the Big Bang’s early state: an initially inhomogeneous region can be smoothed out and made clean by inflation, then expanded into a uniform region larger than our observable universe—and nothing beyond this uniform region’s horizon can mess things up. They also happen to be exactly the effects necessary to create an infinite space from a finite one.
A version of inflation in which this idea is particularly clear is called “open inflation.” In this model, the universe is permeated by a scalar field known as an “inflaton”—a field that determines the density of vacuum energy at each space-time point. With high field values, the vacuum energy is large and drives rapid inflation; with low field values, the energy is low and inflation absent. The normal tendency of such a system would be to evolve from high to low energy, so that inflation would proceed for a while, then end. Imagine, however, that there is an energy barrier to this evolution. The field then gets “stuck” at large values and, as it turns out, only occasionally creates a small bubble in which the field is small. This is physics analogous to that of a carbonated beverage, which is “stuck” in the cola phase but occasionally creates small bubbles in the “bubbly” phase. The key differences are that in open inflation the bubbles expand at essentially the speed of light and the space between the bubbles also expands, even faster than the bubbles themselves.
This situation is depicted in Figure 3. An initially finite region begins to inflate. Soon thereafter, a bubble forms within it and starts to expand at a rate rapidly approaching the speed of light. Yet this bubble expansion cannot catch up with the receding edge of the initial region. Inside the wall of the bubble, the inflaton field relaxes sequentially to lower and lower constant values. Indeed, each of the surfaces in Figure 3 is just the same sort of hyperboloid as the strange dicing of conventional space shown in Figure 2. Just as in that construction, each surface constitutes an infinite, homogeneous space. As such, it is natural to
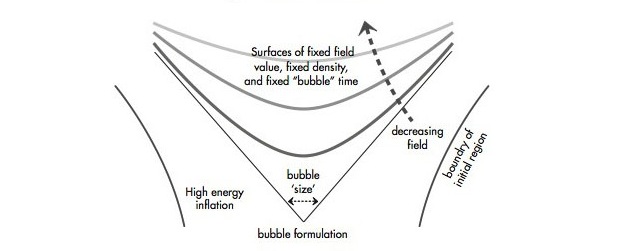
FIGURE 3: The structure of an inflationary “bubble.” Consider an initial inflating region that subsequently expands, as indicated by the outermost gray lines labeled “boundary of initial region.” Within this region a bubble can form, with the border of the bubble growing at the speed of light and thus tracing out a light cone in space-time, as shown by the diagonal lines. Inside this light cone, surfaces upon which the field takes constant values are shown as hyperbolas. Like those in Figure 2, these hyperbolas constitute possible surfaces of constant “bubble” time—but here they are also surfaces of constant density, so in this description the space is of uniform density. As as this “bubble” time increases, the field evolves from high to low energy and the density decreases, until the (possible) end of inflation and the creation of matter and energy that may be termed the Big Bang.
identify these surfaces with fixed times at which the space is progressively less and less curved—as if expanding. In short, in one way of dividing space-time into space and time, the bubble is of finite size and inhomogeneous inside; in another way, the interior of the bubble is infinite, uniform, and expanding.
The scenario depicted in Figure 3 is worth studying, because it is probably contemporary cosmology’s best guess as to how our observable universe formed.
3. The creation of an infinite space probably actually happens—in fact, an infinite number of times.
Consider a small, uniform region suffused with high values of the inflaton field. It will begin to inflate and eventually spawn one bubble, then another, and another. Because the bubbles cannot grow faster than the region from which they were spawned, it can be shown that inflating space endures forever. Despite the bubbles taking “bites” out of it, the space’s physical volume increases exponentially for all time. This process has been dubbed “everlasting” or “eternal” inflation, and it provides a rather different cosmic picture. The Big Bang is not the “beginning of the universe,” just the end of our particular universe’s inflation. And whereas the Big Bang appears to occur everywhere at once from the bubble’s inside perspective, from the outside, the Big Bang—and all that evolves from it—looks like just one of many bubbles that form a sort of “steady state” in the vast, eternally enduring, inflating background.
Is this just a speculative possibility that is allowed by the physics we understand, or do we have reasons to think it actually happened? In fact we do, and they are pretty good reasons.
Although “bubbly” eternal inflation has been described here, there are many different inflation scenarios with different properties and varying levels of connections to other fundamental theories. Most of them are eternal. Beyond explaining puzzling features of the standard Big Bang model (such as the uniformity of space), inflation makes certain predictions, which have been verified. As a recent and impressive example, seven years of data from the Wilkinson Microwave Anisotropy Probe—which looks at the Big Bang’s omnipresent remnant radiation, known as the cosmic microwave background—has confirmed in great detail inflation’s predictions for the particular type of nonuniformities in the early universe. Moreover, string theory, our best current candidate for a true fundamental theory, seems to require eternal inflation. Most string theorists now believe that while the four fundamental forces of nature (electromagnetism, the strong and weak nuclear forces, and gravity) are unified at the highest energies, at low energies they can be separated, but in many different ways. Just like the inflating and noninflating phases of the bubble model, these phases can coexist. Some would drive inflation and others would not, and there can be transitions between the different phases, often manifesting as, yes, bubble formation.
In this picture, dubbed the string theory “landscape,” inflation brings into being an infinite number of sub-universes with a diverse set of properties spanning the array of ways in which low-energy physics can emerge from high-energy unification. Inflation can spawn bubbles inside of which is inflation, which spawns bubbles, and so on. And the incredibly rich structure of this “multiverse” is that of infinitely many times, each forming infinitely many sub-universes, each of which is infinite.
4. This raises profound issues that we cannot ignore.
Infinity can violate our human intuition, which is based on finite systems, and create perplexing philosophical problems. A classic example was invented by the mathematician David Hilbert. Rather than imagining an infinite universe, imagine an infinite hotel, with all rooms completely filled. Though the hotel is full, you can accommodate infinitely many more guests by moving each guest into the room of twice its current number and adding guests in all of the odd rooms. Yet although you double the number of guests, the hotel looks exactly the same. Applying this notion to an eternally inflating universe, suppose the whole bubbling mess is infinite at some time. Although infinitely many bubbles have formed during some time interval, it is rather unclear that after this time the universe is actually any bigger!
The same example reveals problems for such naïve questions as “What is the chance that a randomly chosen guest is in an even-numbered room?” The seemingly obvious answer is 50 percent. Yet the number of even-numbered rooms is just as large as the total number of rooms in the hotel (since each guest found an even-numbered room to go to) and thus twice as large as the odd-numbered rooms (which make up just half of all rooms, remember?) This implies that a randomly chosen guest is twice as likely to be in an even-numbered room. Yet, by exactly the same reasoning, there are as many odd-numbered rooms as total rooms and twice as many as even-numbered rooms! Our intuition that the answer is “obviously” 50 percent arises from considering the first N rooms, then letting N approach infinity. But that’s only one way of comparing the number of even- and odd-numbered rooms, and different choices—that is, different ways of measuring—would give different results.
This “counting” or “measure” ambiguity afflicts infinite systems terribly and creates a nightmare in cosmology. If postinflationary regions can have different properties, and each possible set of properties is realized in an infinite number of such regions, there is a twofold problem. First, there is no unique prediction, from this fundamental theory, for what we can observe. This is a letdown but not fatal, since we would still hope to make probabilistic predictions. Yet the measure ambiguity suggests that the relative probabilities themselves depend on the particular measure we choose and there is no compelling reason to believe any one given measure. This measure problem has spawned a large amount of recent work in inflationary cosmology, and although there has been progress, it’s not clear that the progress is toward any particular resolution.
Another key aspect of infinity is that it is so much larger than anything finite. In particular, an infinite system including some randomness will tend to contain infinitely many realizations of any given finite subsystem compatible with the properties of the infinite one. This means that if we reside in an infinite bubble, then somewhere (incredibly far away) within it is another copy of the entire Earth, perfect in every detail. On a more personal level, there are infinitely many identical copies of you, as well as infinitely many of every possible small or large variation of you, some more common than others. What does this mean? If these other people are identical to you, are they you? What is your relation to them? If you were to suddenly cease to exist, should you take heart that “you” continue merrily along out there? Or would “you” simply find yourself to be one of them? (Beware: This possibility becomes more and more disturbing the more you think about it.)
Should we embrace the idea that our world is truly infinite, or should we look for some way to tame and regulate this infinity in our theories? It is difficult to say. What seems clear, however, is that infinity can no longer be safely ignored: beautifully constructed, empirically supported, self-consistent theories have brought infinity from idle curiosity to central player in contemporary cosmology. And if correct, the worldview these theories represent constitutes a perspective shift unlike any other: in comparison to the universe, we would be not just small but strictly zero. Yet here we are, contemplating—if not quite understanding—it all.
|
Edited by Max Brockman JUST PUBLISHED — AVAILABLE IN BOOKSTORES AND ONLINE — ORDER NOW! amazon.com | bn.com | amazon.co.uk (available October) Advance Praise for Future Science: "This remarkable collection of fluent and fascinating essays reminds me that there is almost nothing as spine-tinglingly exciting as glimpsing a new nugget of knowledge for the first time. These young scientists give us a treasure trove of precious new insights." — Matt Ridley, Author, The Rational Optimist "I would have killed for books like this when I was a student!" — Brian Eno, Composer; Recording Artist; Producer: U2, Cold Play, Talking Heads, Paul Simon "Future Science shares with the world a delightful secret that we academics have been keeping — that despite all the hysteria about how electronic media are dumbing down the next generation, a tidal wave of talent has been flooding into science, making their elders feel like the dumb ones..... It has a wealth of new and exciting ideas, and will help shake up our notions regarding the age, sex, color, and topic clichés of the current public perception of science." — Steven Pinker, Johnstone Family Professor, Department of Psychology, Harvard; Author, The Language Instinct Eighteen original essays by: Kevin P. Hand: "On the Coming Age of Ocean Exploration" Felix Warneken: "Children's Helping Hands" William McEwan: "Molecular Cut and Paste" Anthony Aguirre: "Next Step Infinity" Daniela Kaufer and Darlene Francis: "Nurture, Nature, and the Stress That Is Life" Jon Kleinberg: "What Can Huge Data Sets Teach Us About Society and Ourselves?" Coren Apicella: "On the Universality of Attractiveness" Laurie R. Santos: "To Err Is Primate" Samuel M. McLure: "Our Brains Know Why We Do What We Do" Jennifer Jacquet: "Is Shame Necessary?" Kirsten Bomblies: "Plant Immunity in a Changing World" Asif A. Ghazanfar: "The Emergence of Human Audiovisual Communication" Naomi I. Eisenberger: "Why Rejection Hurts" Joshua Knobe: "Finding the Mind in the Body" Fiery Cushman: "Should the Law Depend on Luck?" Liane Young: "How We Read People's Moral Minds" Daniel Haun: "How Odd I Am!" Joan Y. Chiao: "Where Does Human Diversity Come From?" |





